Test Results: The Lehmer PRNG



Implementation

This version of the Lehmer Generator uses the constants proposed by Park and Miller in their 1988 paper, "Random Number Generators: Good Ones Are Hard to Find". These values were popularized by the work, and provide an idea of how random the earliest versions of the Lehmer Generator were in practice.

These constants are:


This gives the generator a possible range of 0 to 2,147,483,646.


Test Results

Period Length Test
SEEDNumber indicates how many results could be obtained before the generator fell into a loop.
1138-PASS
65535-PASS
8675309-PASS
16777216-PASS
123456789-PASS


Dice Roll Test
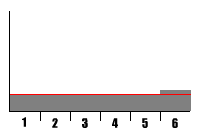This generator produced an ideal distribution of outcomes.


Dartboard Test
SEEDMinimum of 6,000 darts placed needed to pass.
11387015PASS
655357000PASS
86753096979PASS
167772166969PASS
1234567896930PASS


Crush Test
SEED70% or higher required to pass.
1138101%PASS
65535101%PASS
8675309101%PASS
16777216101%PASS
123456789101%PASS


Plot Test
An ideal result for this test.


Example Output
192451401420558258311400345034132569896587966713012880279621250795574403791735479365625148689947852326607113247222631313902115925907974373489711835115563627129127309498013527711457138995689
178582073410576258667957836432075343855169659672075655254181349211012649089920712126101604184001060071815110265919317566667886935269601713864451721670746132589766149565522311812448261842957714
14336585177321758795987010431407543506205133363710619681217616194301535223890491900525170756637296555696146142918714698751701687590749152119251488976526313561411801420866649487615103549439169
2444312832035667068465281774251469341697253481823877718013332981931767727156241234334213285164454724617910266325063240251457678761713491151970900091851693190744320061143242288936944707
187959074579720384545454928210104501953357468891458422754332879620512872905200303892411215452961347820153115580291515900052902079889409210997484494974019737542828176960162511934840721389535124
321679431628222604153191707200761044364565383726917016813424529941144274776111010134713541389317161174782081073536536760863190850704114620864951779832491134495717428235509617507222491715391396
615231597636904749989403121626715382738565356493282241895050361781448670194529528512868130671594081321260366715204684997201942573216567205362290815501882915426840542590851880164276441799
115018733216981822771291860909124662639311993270198076975917292792501307181321103875323714310877964757409726968986233993460239222116861222326203807926619283585552956159771557140696834976752
17961213662341724831546880477983146357100164208147414653418164665687260826241268579314794882982102510487611870115154932096911983061088291155902071819394176870250011142757261574725042815315266
205800780215655096325507419806659392901904362513524481103168101083345421029917556138551767512056876186341196877131434619268105415382942331625362941660129852529615858290586094348891422666880
6793264621416779382512395338419021296892043359989395006795987121147990548461587003456482898120294712101111275773400818813295473481134930801832219753556395760119973928212941508911079648221
15583168442068121943190266930621315221121699371302127577047437222732186090033720012905160956895515284304951319440511380541453134687838335986005485397762611687472811026326585177144651770759258
132246908028208111014408068416149731151435079467562629415050815696960521701205395981187701051638096798212801217671515362323168599288843574645167136568776402007110806078845075037091957274926
76517653611899623161814405014372156738896929546671959715459879351014578492100055786415790642387297384404348530636885791001595600171665614263152957959611753173518183985529846830071058314867
163840521516411266711359974297861887958197574337672810161512229611671307928515894461832795314285409830155202905916160181511191380248390303508141600101831533347219675067559328347791523507553
11439200901591344686968797864364688694404551520364170238280796116131874915338293784150601723513894051032141872290179203369109894968916880588237981776441788803546179762326918543360871543928945
76887191410211545992003522216654299352169293642411716390651460206112241006468441549434156533685319418126217357376883324827902858020361701384360142217871510646718951106792461357541713544326085
208174375535859662179440736314916951201195786762143614030816414479231240312499308409264155645983792217635262846766513314697091231797423107693128199186285114708687431206702984221489820990244489
408623731726619618349397815110878580716474532301239775839202618287914074568745953096132423543181620027914201147393211548040501981950411102170906058493000818859921371011216839335830715719802689
948410472131117487015863382235770364562155661402152024915458046891436751686117945973419056875281319171738698228938128311375826214753214246103271130585486814954546290354056897773208654203034


A WFTID Website